Queries about input and output files, running specific calculations, etc.
Moderators: Global Moderator, Moderator
-
hszhao.cn@gmail.com
- Full Member

- Posts: 191
- Joined: Tue Oct 13, 2020 11:32 pm
#1
Post
by hszhao.cn@gmail.com » Wed Mar 30, 2022 9:07 am
I noticed the following description given by
pyprocar documentation:

OTOH, I also noticed the following description on
vaspwiki:

In case of non-collinear calculations, it seems that the forms of the two equations mentioned above do not correspond exactly.
Any tips/comments on the differences and connections between them will be highly appreciated?
Regards,
HZ
-
marie-therese.huebsch
- Full Member

- Posts: 212
- Joined: Tue Jan 19, 2021 12:01 am
#2
Post
by marie-therese.huebsch » Wed Mar 30, 2022 9:24 am
Hi HZ,
Could you please clarify your question? In particular, can you specify what you mean by "it seems that the forms of the two equations mentioned above do not correspond exactly"?
Cheers,
Marie-Therese
-
hszhao.cn@gmail.com
- Full Member

- Posts: 191
- Joined: Tue Oct 13, 2020 11:32 pm
#3
Post
by hszhao.cn@gmail.com » Wed Mar 30, 2022 9:42 am
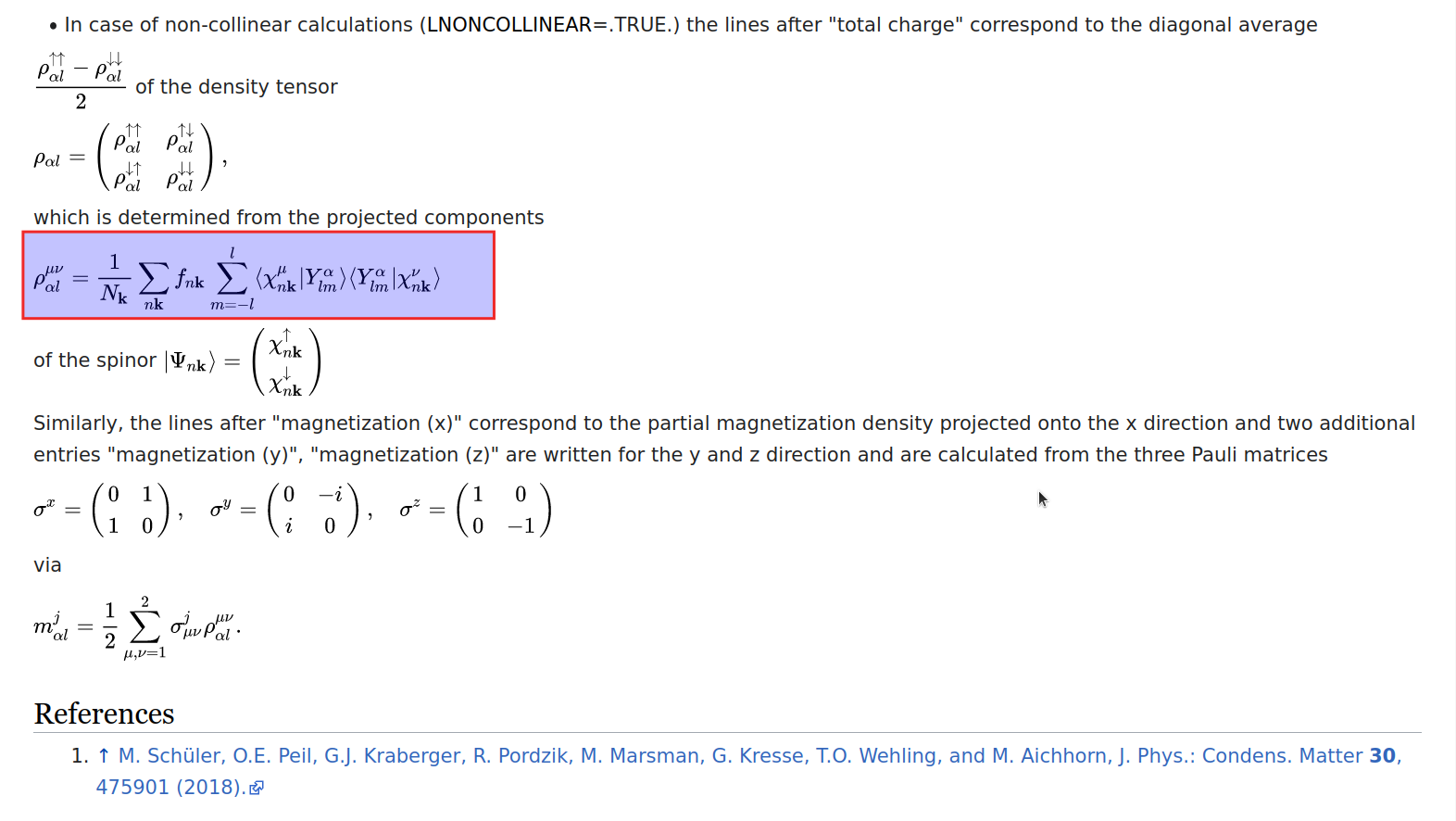
I mean whether the two equations in the red box below are equivalent and can be derived from each other:
1. The version used by pyprocar:
 2. The version described by vaspwiki:
2. The version described by vaspwiki:
-
marie-therese.huebsch
- Full Member

- Posts: 212
- Joined: Tue Jan 19, 2021 12:01 am
#4
Post
by marie-therese.huebsch » Wed Mar 30, 2022 11:40 am
Well, the first red box is not an equation.
If you are wondering about the sum over k points, it is required as the projected charge density is given in real space. fnk is the occupation number, to show that the sum is over occupied states.
The second red box are the projected components. To obtain the magnetization as a vector, look at the definition of m^j_alpha,l below. If you were to sum over all k points in the first red box, then it equals to m^i_alpha,l.
Best regards,
Marie-Therese




